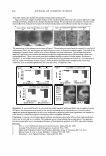
160 JOURNAL OF COSMETIC SCIENCE where k is the Boltzmann constant, T is the absolute temperature, V 1 is the molar volume of the solvent, and N av is the Avogadros's constant. X is a dimensionless quantity that gives a measure of the polymer-solvent interaction, i.e., the solvation of the A chains by the molecules of the medium. It is referred to as the Floury-Huggins inter- action parameter. It is clear from equation 2 that when the Flory-Huggins interaction parameter, X, is less than 0.5, the chains are in good solvent conditions, Gmix is positive, and the interaction is repulsive and increases very rapidly with decreasing h, when the latter is lower than 28. This explains why the hydrophobically modified inulin (HMI) polymeric surfactant is ideal for stabilizing 0/W emulsions. In this case the polyfructose loops are strongly hydrated by water molecules. For stabilization of W /0 emulsions, the stabilizing chains have to be soluble in the oil phase (normally a hydrocarbon). In this case, poly(hydroxy- stearic acid) (PHS) chains are ideal. A polymeric surfactant of PHS-PEO-PHS (Arlacel P135) is an ideal W/0 emulsifier. Equation 2 also shows that when X 0.5, i.e., when the solvency of the medium for the chains becomes poor, Gmix is negative and the interaction becomes attractive. The condition X = 0.5 is referred to as 0-solvent, in which case mixing of the chains with the solvent does not lead to an increase or decrease of the free energy of the system (i.e., polymer mixing behaves as ideal). The 0-condition denotes the onset of change of repulsion to attraction. Thus, to ensure steric stabilization by the above mechanism, one has to ensure that the chains are kept in better than a 0-solvent. The second repulsive force arises from the loss of configurational entropy when the chains overlap. This is schematically illustrated in Figure 7, whereby the polymer chain is represented by a simple rod with one attachment point to the surface. When the two surfaces are separated at infinite distance, each chain will have a number of configura- tions, 000, that are determined by the volume of the hemisphere swept by the rod. When the two surfaces approach a distance, h, that is smaller than the radius of the hemisphere swept by the rod, the volume available to the chains becomes smaller and this results in a reduction in the configurational entropy to a value, D (which is less than 000). This results in strong repulsion, and the effect is referred to as entropic, volume restriction or elastic repulsion, and is given by the following expression (8): - - - - hoo no. of configurations (loo n Gel = 2v ln noo Figure 7. Schematic representation of the entropic, volume restriction or elastic interaction. (3) lost

EMULSION STABILIZATION 161 where v is the number of polymer chains per unit area of the surface. It should be mentioned that G el is always repulsive and becomes very high on considerable overlap of the polymer chains. Plots of G m ix and G el versus h are illustrated in Figure 8. This figure shows that G m ix increases very rapidly with a decrease in h as soon as h becomes smaller than 28 (and X 0.5). G el also increases very rapidly with a decrease in h on further overlap. Combi- nation of G mix ' G e1 , and G A (the van der Waals attraction) results in the total Gr - h curve shown in Figure 8. This curve shows a minimum (G mi n) at h - 28, but when h 28, Gr increases very rapidly with a further decrease in h. The depth of the minimum, G min ' depends on the adsorbed layer thickness. With an increase of 8, G min decreases, and at sufficiently high values of 8 (of the order of 5-10 nm), it reaches small values (fraction of kT units). This shows that with sterically stabilized dispersions, there is only weak attraction at relatively long distances of separation, which in most cases is over- come by Brownian diffusion. Thus, one can say that the net interaction is repulsive, and this ensures the long-term stability of the emulsion. From the above discussion one can summarize the main criteria for effective steric stabilization. First, there should be enough polymer to ensure complete coverage of the surface by the chains. This will prevent any attraction between the bare patches or bridging by the polymer chains (which can adsorb simultaneously on more than one particle). Secondly, the chains must be strongly adsorbed ("anchored") to the surface. This prevents any displacement on close approach. In this respect, block and graft copolymers containing an anchoring chain (such as the alkyl groups of HMI) for oil droplets are the best stabilizers. The third criteria for effective steric stabilization is to ensure that the stabilizing A chain remains in good solvent condition at all times and G h h Gmin Figure 8. Schematic representation of the variation of Gmix' Ge1, GA, and GT with b.