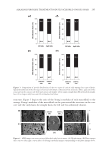
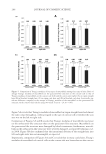
EFFECT OF ISOFERULIC ACID ON MUSHROOM TYROSINASE 239 The kinetic parameters of Km and vm for mushroom tyrosinase were calculated from the Lineweaver–Burk plot, using a method introduced by Huang et al. (5). The equilibrium constant KI, in the binding and dissociation equilibrium of the inhibitor and the enzyme, was obtained from the linear plot of Km versus cI, as shown in Figure 6. The inhibition constant of isoferulic acid obtained from the experiment data is also listed in Table I. Figure 4. Effect of concentration of isoferulic acid on diphenolase inhibition rate. Figure 5. Lineweaver–Burk plot of isoferulic acid inhibitory effect on diphenolase activity of tyrosinase.

JOURNAL OF COSMETIC SCIENCE 240 Table I Kinetic Parameters of the Isoferulic Acid Inhibitory Effect on Diphenolase Activity cI (mmol/L) Michaelis–Menten equation Km (mmol/L) vm (μM/min) KI (mmol/L) 0 1/v = 0.023/cS + 0.039 0.59 64.5 0.11 0.20 1/v = 0.071/cS + 0.040 1.79 0.40 1/v = 0.111/cS + 0.039 2.84 CONCLUSIONS Described in this paper is a detailed investigation on the inhibition kinetics of isoferulic acid on both monophenolase and diphenolase activities of mushroom tyrosinase. The re- sults showed that isoferulic acid is an effective inhibitor against both monophenolase and diphenolase activities of mushroom tyrosinase. Additionally, the presence of isoferulic acid prolongs the lag period in the inhibited monophenol oxidation. Furthermore, a com- petitive inhibition behavior was observed for isoferulic acid, when it was inhibiting the diphenolase activity of mushroom tyrosinase. Concentrations of isoferulic acid leading to 50% rate inhibition (IC50) on monophenolase and diphenolase activity were calculated to be 0.13 mmol/L and 0.39 mmol/L, respectively, which are much lower than that of arbu- tin (IC50 = 5.3 mmol/L for diphenolase activity). ACKNOWLEDGMENTS This work was fi nancially supported by High Level Teacher Program of Guangdong Province, Science Program of Guangzhou City, and Science Program of Chancheng Dis- trict of Foshan City in Guangdong Province. Dr. Keliang Pang is gratefully thanked for his help in editing this paper. Figure 6. Effect of isoferulic acid concentration on Michaelis constant (Km).